OEF vector subspaces
--- Introduction ---
This module gathers for the time being 8 exercises on
subspaces of vector spaces.
Dimension of intersection
Fill-in: Let F be a vector space of dimension , and let
,
be two vector subspaces of F, of dimensions respectively and . Then
is at least
and at most
.
Dim subspace by system
Let E be a sub-vector space of R defined by a homogeneous linear system. This system is composed of equations, and the rank of the coefficient matrix of this system is equal to . What is the dimension of E?
Dimension of sum
Fill-in: Let F be a vector space of dimension , and let
,
be two vector subspaces of F, of dimensions respectively and . Then
is at least
and at most
.
Subbase
Fill-in: let F be a vector space of dimension , and let B be a basis of F. Let
be a subset of de elements, and let E be the vector subspace of F generated by
. Then dim(E) is
equal to
.
Subbase II
Fill-in: let F be a vector space of dimension , and let B be a basis of F. Let
and
be two subsets of B, with respectively and elements. Suppose that
has elements. Let
and
be the vector subspaces of F generated respectively by
and
, and let
.
Then
is
equal to
.
Dimension of subspace
Fill-in: Let E be a vector subspace of 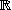 . Then dim(E) is
equal to
.
. Then dim(E) is
equal to
.
Dim subspace of matrices
Fill-in: let M× be the vector space over 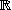 of × matrices, and let E be the vector subspace of M× consisting of matrices A such that =0, where B is a fixed non-zero matrix of dimension ×. Then dim(E) is at least
, and at most
.
of × matrices, and let E be the vector subspace of M× consisting of matrices A such that =0, where B is a fixed non-zero matrix of dimension ×. Then dim(E) is at least
, and at most
.
Extension of subspace
Let F be a vector space of dimension , E a subspace of F generated by a set S, with
. Let v be a vector of F which a linear combination of vectors in S, and let
be the vector subspace of F generated by
. What is the dimension of
?
This page is not in its usual appearance because WIMS is unable to recognize your
web browser.
Please take note that WIMS pages are interactively generated; they are not ordinary
HTML files. They must be used interactively ONLINE. It is useless
for you to gather them through a robot program.
- Description: collection of exercises on vector subspaces. This is the main site of WIMS (WWW Interactive Multipurpose Server): interactive exercises, online calculators and plotters, mathematical recreation and games
- Keywords: wims, mathematics, mathematical, math, maths, interactive mathematics, interactive math, interactive maths, mathematic, online, calculator, graphing, exercise, exercice, puzzle, calculus, K-12, algebra, mathématique, interactive, interactive mathematics, interactive mathematical, interactive math, interactive maths, mathematical education, enseignement mathématique, mathematics teaching, teaching mathematics, algebra, geometry, calculus, function, curve, surface, graphing, virtual class, virtual classes, virtual classroom, virtual classrooms, interactive documents, interactive document, algebra, linear algebra, vector space, subspace, base, dimension, linear system
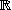 . Then dim(E) is
equal to
.
. Then dim(E) is
equal to
.
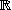 of × matrices, and let E be the vector subspace of M× consisting of matrices A such that =0, where B is a fixed non-zero matrix of dimension ×. Then dim(E) is at least
, and at most
.
of × matrices, and let E be the vector subspace of M× consisting of matrices A such that =0, where B is a fixed non-zero matrix of dimension ×. Then dim(E) is at least
, and at most
.